Section 1.2, Problem 15
Solve
(x ln x) y' = y
Separating the variables gives:Integrating both sides gives:
For the right hand side, we perform a change of variable with u = ln x, du = dx / x and get:
ln | y | = ln | u | + C
Substitution ln x for u gives:
ln | y | = ln | ln | x | | + C
Taking e to the power of both sides gives:
y = C ln | x |
Section 1.2, Problem 16
SolveSeparating the variables gives
Integrating both sides gives:
Section 1.2, Problem 17
Solve
y' sin 2x = y cos 2x
Separating the variables gives:Integrating both sides gives:
ln | y | = ln | sin 2x | + C
Taking e to the power of both sides gives
y = C sin 2x



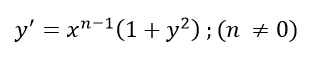






No comments:
Post a Comment